WEEK 11

6.2 Perimeter, area and volume 

 

6..2.1 Understand the concept of perimeter (3)
6..2.2 Understand the concept of area (4)

As with Week 8, here we look at the perimeter and area of a set of 'dynamic' shapes, and we consider what happens to the perimeter and area as the shapes change in some way.

Monday: In this task, the 'U-shape' changes in an interesting way and it is worth getting students to spend some time trying to visualise it. The shape starts as a 12-by-12 square (when e = 0) and then begins to 'hollow out' and look more like a letter U, before closing-up again. Expressing the perimeter algebraically is a way of getting more insight into how it changes, and might demonstrate to students the power that algebraic symbolisation can have.

As students visualise the shape as it changes (or as they perhaps try specific values of e) they might notice that the total length of the horizontal edges at the top of the shape is always 12 units. The perimeter does change, however, because of the two vertical edges of length e. So it increases, from a value of 48 units when e = 0, to 48+12=60 units as e approaches 6 (it could be argued that when e = 6, the two vertical edges of length e 'disappear' and so the perimeter snaps back to 48 units).

Some students might express the perimeter, p, as p = 12 + 12 + 12 + e + e + (12 – e – e) + e.
Others might go directly for p = 48 + 2e. Both forms are illuminating, though the simplified version is perhaps more powerful in helping to show how and why the perimeter changes.

An animation of a similar task can be found here:  N-shape.

Tuesday: Here we encounter Monday's dynamic shape again, but this time consider its area. The area changes in a more complex way than the perimeter, which is why we have scaffolded the task by asking about a specific area. You might want to hide this scaffolding initially, to give students the opportunity to cope without it....

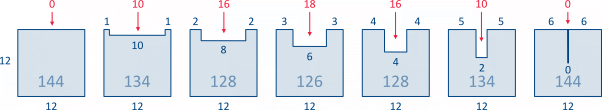
How readily do students see that the area decreases and then increases again as e goes from 0 to 6? A useful way to visualise the changing shape is to see it as a square (of area 144 square units) with a white rectangular piece taken out. This rectangle starts off as long-and-thin, becomes more square-like and then more thin-and-long. Not surprisingly, this rectangle has a maximum area when the value of e is half-way between 0 and 6. It may come as a surprise, however, that the rectangle is not a square at this point. In fact it is a 'half-square', a shape that we encountered in the Friday task of Week 9.

It is worth asking students to draw the shape for different values of e, as here. We can see that the area has a maximum value (in unit squares) of 144 when e = 0 and e = 6, a minimum of 126 when e = 3, the value 128 when e = 2 and e = 4, and that the white 'gap' is a square when e = 4.

As with Monday's task, it can be illuminating to express the situation algebraically, though this might be rather demanding for Key Stage 3 students. We can write the area, A,
as this: A = 144 – e(12–2e)
or this: A = 2e² – 12e + 144.
The graph of this is a parabola, albeit a very tall, thin-looking one, with a minimum for A when e = 3.

Wednesday: Here we look at the changing perimeter and area of a dynamic L-shape. Again, it is worth trying to visualise the shape as the variable, e, goes from 0 to 12. The perimeter remains the same but the area changes. The shape is a 12-by-12 square when e = 0 and 12 and so has a maximum area for these values. Its minimum comes halfway between, when e = 6.

The diagram below shows what the L-shape looks like when e has the values 0, 2, 4, 6, 8, 10 and 12. Notice how the white rectangle that has been removed from the 12-by-12 square is a square this time when the L-shape's area is a minimum. Notice, too that for each white rectangle of size a-by-b there is a another of size b-by-a.

 

Thursday: Here we consider another, slightly more complex L-shape. Again, the perimeter stays the same but the area changes - this time in a less 'symmetrical' way.

The diagram below shows what the L-shape looks like when e has the values 0, 2, 4, 5, 6, 7 and 8. Notice how the white rectangle that has been removed is not a square when the L-shape's area is a minimum (when e = 6). Notice too that there are some pairs of rectangles with the same area, though their dimensions are different, for example, 6-by-8 and 12-by-4. This difference might seem surprising at first, especially in the case of the 7.5-by-7 and 10.5-by-5 pair of rectangles!
[How obvious is it that 7½×7 must equal 10½×5?]

 

Friday: We can think of the U-shape as an honorary L, by pushing the top left e-by-e (in this case 3-by-3) piece to the right, to join the other e-by-e piece, and then rotating the whole shape 90˚ clockwise. What do the shapes have in common when their areas are a minimum?

If we think of the L-shapes as a square with a white rectangle removed, then it turns out that each has a minimum area when the bottom edge of the white rectangle is 6 cm (ie half of 12 cm) above the base. Will this happen with every L-shape of the sort shown in the diagram below? (In our three L-shapes, k is effectively 0.5, 1 and 1.5 respectively.)